CowPi Journal

Time and Space are not the ultimate reality.

Let’s set the stage with a simple story. In a marvelous little book by Edwin Abbott called Flatland, the main character is a geometric figure called A. Square. He is a mathematician and inhabits a place called Flatland, a two-dimensional plane or flat surface not unlike the top of a table. All of the creatures of Flatland are geometric shapes and live within this plane. Because they are two-dimensional, they do not have the concept of above or below. To them, above means north and below means south.

Imagine for a moment what things might look like if you lived in two-dimensions. If I asked you what shape a coin is, you probably would say and imagine a circle. But you are referencing the circle from three-dimensions. You can look from above the coin/circle. A Flatlander does not see from “above.” They see things from the side. So, now imagine a coin sitting on top of a table. As you lower your eye to the table top, the circle of the coin’s shape appears to look flatter and flatter. Eventually, when your eye is in the plane of the coin, the circle will no longer look like a circle but a line. All sense of “depth” and perspective disappear. Your vision becomes limited. All shapes in Flatland look like a line. You are then forced to infer the shapes of objects by other means, usually by touch. Now you can begin to “see” like a Flatlander.

The first part of the novel describes what life is like as a two-dimensional figure, and how Square’s rigid, class-structured society functions and operates. In the second part, Square is visited by a Sphere from three-dimensional Spaceland. (This novel works on many different levels. It is a cute story. It is a scathing satire on Victorian English society. It explores the mathematical relationships between dimensions. And all of it is wrapped in subtle religious undertones.)

When Sphere first visits Flatland, Square just hears a voice. It seems to come from within his head (as far as it goes that a square has a head), but not really. We as inhabitants of Spaceland know that Sphere is talking to him from above. Remember, Square has no concept of above. Then Sphere moves over and begins to break the surface of the plane. From Square’s point of view, the Sphere begins as a point, then as a circle that grows in size, then a circle that shrinks in size, then to a point again, and finally disappears.

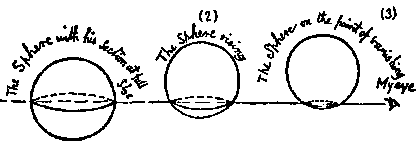
When Sphere re-enters the plane of Flatland at a different place, Square is astonished to see how Sphere could move from one place to another without moving within the “space” of Flatland. Then Square misidentifies Sphere as a Circle, a member of the highest, priestly class of Flatland. Actually, Flatland circles are not true circles. They are many-sided polygons on the order of 200 to 300 sides. (Each succeeding generation has a side added. Square’s sons are pentagons, and his grandsons are hexagons. Therefore, the so-called circles were the blue bloods of Flatland society with their family lines going back many generations.) Sphere appeared as a “perfect” circle with not the hint of an angle or straight edge. Square was honored to be visited by such a creature. (Note: To Square, Sphere appeared as a circle. But in reality, the Sphere was so much more than just a circle.)

Sphere’s mission was to illuminate to an inhabitant of Flatland (an event that occurs every one thousand years) to the higher natures of Spaceland. In vain, the Sphere tried to describe three-dimensions using terms like up and down, above and below, width and length and depth. Poor Square tried his best but could not understand without “seeing” it. Finally, Sphere grabbed Square’s corner and pulled him out and above the plane of Flatland. Square was amazed to see all of Flatland below him. He could see inside his house. He could see into his neighbor’s house. He even could see inside all of the polygons. He could now see what a square truly looks like, inside and out.

The rest of the story is about visiting Spaceland. They visit one-dimensional Lineland and zero-dimensional Pointland too. Square eventually irritates Sphere when he asks to visit four-dimensional land so that he could see the “insides” of Sphere. (This kind of grossed out Sphere.) In the end, when Square returns to Flatland, he is frustrated and persecuted for telling tales of a mysterious three-dimensional place called Spaceland.

I hope this little synopsis of the story of Flatland lays enough of the ground work to ponder a few points:

• Square had to infer his shape as a square. He had never seen the shape of a square, or a pentagon, or any shape for that matter. He did not know his true identity, his true essence until Sphere lifted him above his plane of existence and he could see the reality of Flatland. In fact, Square never directly sees his own true self. It is reflected back to him in Sphere’s descriptions and in seeing other squares from above.

• When Square asked to move to a higher dimension so that he could see the “insides” of Sphere, to see Sphere’s true shape, Sphere reacted negatively by denying the existence of such a higher dimension. (Not unlike the reaction Square receives when he returns to Flatland.) Later, Sphere admits to Square in a dream that there is such a fourth dimension and that every one thousand years, an inhabitant in Spaceland is visited by a fourth-dimensional creature.

• It is easy for Sphere to affect a dream or a voice inside of Square since he can move in a higher dimension but still manipulate objects within the plane of Flatland, even manipulate the inside of Square’s body and mind. Sphere could not do this without Square being aware of it while Square was in Spaceland.

• Keep in mind that from above the plane of Flatland, all of Flatland—its inhabitants, their houses, their cities and country side, everything—can be seen. Nothing is hidden. This includes seeing the true form of the Flatland creatures from the outside, but also their “insides” too.

• Dimensions are not separate worlds or universes. They are not separate dualities or mutually exclusive domains. They do not partially overlap each other either. Each dimension is contained wholely within the larger one. What is true in one-dimension holds true for two-dimensions, but more so. Likewise with two-dimensions to three, and three-dimensions to higher dimensions.

Now, I hope upon reflection, the religious implications of this story may begin to become evident. How can we extrapolate this story to us, to God, to time, prayer and the Body of Christ?

Until Part 2…